For two vectors A and B, |A + B| = |A − B| is always true when
|A| = |B| ≠ 0
A ⊥ B
|A| = |B| ≠ 0 and A and B are parallel or anti-parallel
when either |A| or |B| is zero
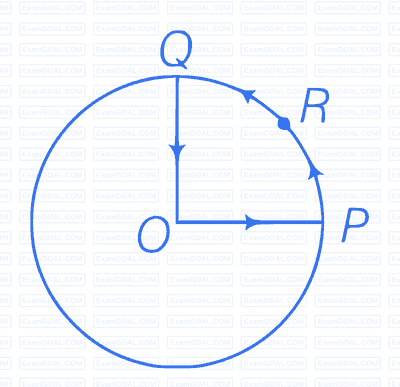
A cyclist starts from centre O of a circular park of radius 1 km and moves along the path OPRQO as shown in figure. If he maintains constant speed of $10 \, \text{ms}^{-1}$, what is his acceleration at point R in magnitude and direction?

As shown in the adjacent figure, the cyclist covers the path OPRQO. As we know whenever an object performing circular motion, acceleration is called centripetal acceleration and is always directed towards the centre.
Hence, acceleration at R is $a = \frac{v^2}{r}$
⇒ $a = \frac{(10)^2}{1 \, \text{km}} = \frac{100}{10^3} = 0.1 \, \text{m/s}^2$ along RO.
A particle is projected in air at some angle to the horizontal, moves along parabola as shown in figure where x and y indicate horizontal and vertical directions, respectively. Shown in the diagram, direction of velocity and acceleration at points A, B and C.
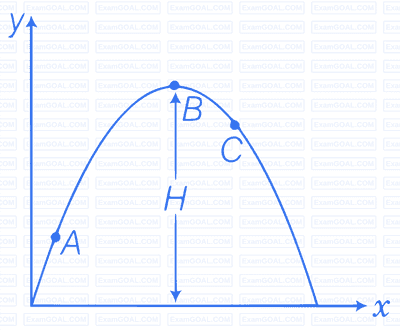
Consider the adjacent diagram in which a particle is projected at an angle $ \theta $.

$ v_x = \text{Horizontal component of velocity} = v \cos \theta = \text{constant.} $
$ v_y = \text{Vertical component of velocity} = v \sin \theta $
Velocity will always be tangential to the curve in the direction of motion and acceleration is always vertically downward and is equal to $ g $ (acceleration due to gravity).
A ball is thrown from a roof top at an angle of 45° above the horizontal. It hits the ground a few seconds later. At what point during its motion, does the ball have
(a) greatest speed
(b) smallest speed
(c) greatest acceleration
Explain.

Consider the adjacent diagram in which a ball is projected from point $ O $, and covering the path $ OABC $.
(a) At point $ B $, it will gain the same speed $ u $ and after that speed increases and will be maximum just before reaching $ C $.
(b) During upward journey from $ O $ to $ A $ speed decreases and will be minimum at point $ A $.
(c) Acceleration is always constant throughout the journey and is vertically downward equal to $ g $.
A football is kicked into the air vertically upwards. What is its (a) acceleration and (b) velocity at the highest point?
(a) Consider the adjacent diagram in which a football is kicked into the air vertically upwards. Acceleration of the football will always be vertical downward and is equal to g.
(b) When the football reaches the highest point velocity will be zero as it is continuously retarded by acceleration due to gravity g.