Three vectors A, B and C add up to zero. Find which is false.
(A × B) × C is not zero unless B, C are parallel
(A × B) . C is not zero unless B, C are parallel
If A, B, C define a plane, (A × B) × C is in that plane
(A × B) . C = |A| |B| |C| → C² = A² + B²
It is found that |A + B| = |A|. This necessarily implies.
B = 0
A, B are antiparallel
A, B are perpendicular
A · B ≤ 0
Two particles are projected in air with speed $v_0$ at angles $\theta_1$ and $\theta_2$ (both acute) to the horizontal, respectively. If the height reached by the first particle is greater than that of the second, then tick the right choices.
Angle of projection $q_1 > q_2$
Time of flight $T_1 > T_2$
Horizontal range $R_1 > R_2$
Total energy $U_1 > U_2$
A particle slides down a frictionless parabolic $(y + x^2)$ track $A – B – C$ starting from rest at point A (figure). Point B is at the vertex of parabola and point C is at a height less than that of point A. After C, the particle moves freely in air as a projectile. If the particle reaches highest point at P, then
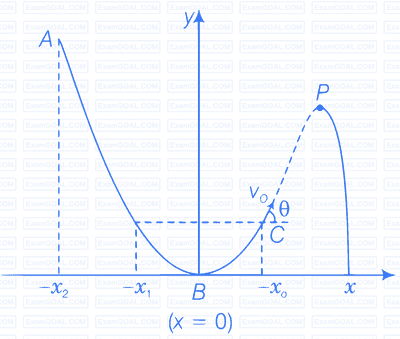
KE at P = KE at B
height at P = height at A
total energy at P = total energy at A
time of travel from A to B = time of travel from B to P
Following are four different relations about displacement, velocity and acceleration for the motion of a particle in general. Choose the incorrect one(s).
$v_{av} = \frac{1}{2}\left[ v(t_1) + v(t_2) \right]$
$v_{av} = \frac{r(t_2) - r(t_1)}{t_2 - t_1}$
$r = \frac{1}{2} \left( v(t_2) - v(t_1) \right) (t_2 - t_1)$
$a_{av} = \frac{v(t_2) - v(t_1)}{t_2 - t_1}$