A, B and C are three non-collinear, non co-planar vectors. What can you say about direction of $A \times (B \times C)$?
The direction of $(\mathbf{B} \times \mathbf{C})$ will be perpendicular to the plane containing $\mathbf{B}$ and $\mathbf{C}$ by right hand rule. $\mathbf{A} \times (\mathbf{B} \times \mathbf{C})$ will lie in the plane of $\mathbf{B}$ and $\mathbf{C}$ and is perpendicular to vector $\mathbf{A}$.
A boy travelling in an open car moving on a levelled road with constant speed tosses a ball vertically up in the air and catches it back. Sketch the motion of the ball as observed by a boy standing on the footpath. Give explanation to support your diagram.
The path of the ball observed by a boy standing on the footpath is parabolic. The horizontal speed of the ball is same as that of the car, therefore, ball as well as car travels equal horizontal distance. Due to its vertical speed, the ball follows a parabolic path.

Note: We must be very clear that we are working with respect to ground. When we observe with respect to the car motion, it will be along the vertical direction only.
A boy throws a ball in air at 60° to the horizontal along a road with a speed of 10 m/s (36 km/h). Another boy sitting in a passing by car observes the ball. Sketch the motion of the ball as observed by the boy in the car, if car has a speed of (18 km/h). Give explanation to support your diagram.
Consider the diagram below

The boy throws the ball at an angle of 60°.
∴ Horizontal component of velocity = 4 cos θ
$= (10 \, \text{m/s}) \cos 60° = 10 \times \frac{1}{2} = 5 \, \text{m/s}.$
Speed of the car = 18 km/h = 5 m/s.
As horizontal speed of ball and car is same, hence relative velocity of car and ball in the horizontal direction will be zero.
Only vertical motion of the ball will be seen by the boy in the car, as shown in fig. (b)
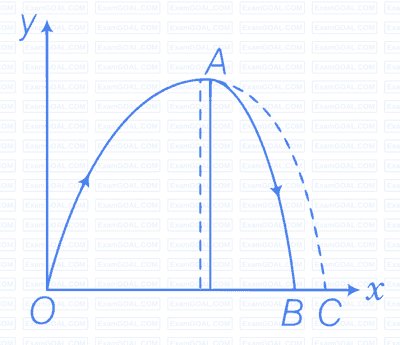
In dealing with motion of projectile in air, we ignore effect of air resistance on motion. This gives trajectory as a parabola as you have studied. What would the trajectory look like if air resistance is included? Sketch such a trajectory and explain why you have drawn it that way.
Due to air resistance, particle energy as well as horizontal component of velocity keep on decreasing making the fall steeper than rise as shown in the figure.
When we are neglecting air resistance path was symmetric parabola (OAB). When air resistance is considered path is asymmetric parabola (OAC).
A fighter plane is flying horizontally at an altitude of 1.5 km with speed 720 km/h. At what angle of sight (w.r.t. horizontal) when the target is seen, should the pilot drop the bomb in order to attack the target?
Consider the adjacent diagram. Let a fighter plane, when it be at position $ P $, drops a bomb to hit a target $ T $.
Let
$ \angle P'\,PT = \theta $
Speed of the plane $ = 720\, \text{km/h} = 720 \times \frac{5}{18} \text{ m/s} = 200 \text{ m/s} $
Altitude of the plane $(P'T) = 1.5\, \text{km} = 1500\, \text{m} $
If bomb hits the target after time $ t $, then horizontal distance travelled by the bomb,
$ PP' = u \times t = 200t \hspace{30mm} \text{...(i)}$
Vertical distance travelled by the bomb,
$ P'T = \frac{1}{2} gt^2 \implies 1500 = \frac{1}{2} \times 9.8t^2 $
$ \implies t^2 = \frac{1500}{4.9} \implies t = \sqrt{\frac{1500}{49}} = 17.49\, \text{s} $
Using value of $ t $ in Eq. (i),
$ PP' = 200 \times 17.49\, \text{m} $
Now,
$ \tan \theta = \frac{P'T}{P'P} = \frac{1500}{200 \times 17.49} = 0.49287 = \tan 23^\circ 12' $
$ \theta = 23^\circ 12' $
Note Angle is with respect to target. As seen by observer in the plane motion of the bomb will be vertically downward below the plane.