Refer to the graph in figure. Match the following:
| Graph | Characteristics |
|---|---|
| (a) | (i) has v > 0 and a < 0 throughout |
| (b) | (ii) has x > 0 throughout and has a point with v = 0 and a point with a = 0 |
| (c) | (iii) has a point with zero displacement for t > 0 |
| (d) | (iv) has v < 0 and a > 0 |

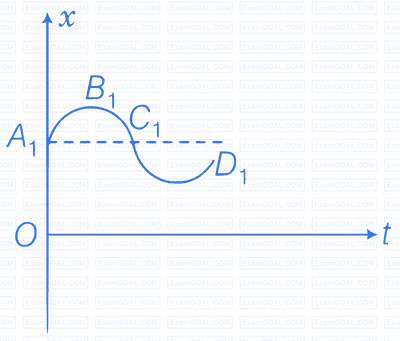


We have to analyze slope of each curve i.e., $\frac{dx}{dt}$. For peak points $\frac{dx}{dt}$ will be zero as x is maximum at peak points.
For graph (a), there is a point (B) for which displacement is zero. So, a matches with (iii)
In graph (b), x is positive (> 0) throughout and at point $B_1$, $v = \frac{dx}{dt}$ = 0. since, at point of curvature changes a = 0, So b matches with (ii)
In graph (c), slope $V = \frac{dx}{dt}$ is negative hence, velocity will be negative. so matches with (iv)
In graph (d), as slope $V = \frac{dx}{dt}$ is positive hence, V > 0
Hence, d matches with (i)
A uniformly moving cricket ball is turned back by hitting it with a bat for a very short time interval. Show the variation of its acceleration with time (Take acceleration in the backward direction as positive).
If gravity effect is neglected then ball moving uniformly turned back with the same speed when a bat hit it. Acceleration of the ball is zero just before it strikes the bat. When the ball strikes the bat, it gets accelerated due to the applied impulsive force by the bat.
The variation of acceleration with time is shown in graph

Give examples of a one-dimensional motion where
(a) the particle moving along positive x-direction comes to rest periodically and moves forward.
(b) the particle moving along positive x-direction comes to rest periodically and moves backward.
When we are writing an equation belonging to periodic nature it will involve sine or cosine function.
(a) The particle will be moving along positive x-direction only if t > sin t
Hence,
x(t) = 1 - sin t
Velocity v(t) = $\frac{dx(t)}{dt}$
v(t) = 1 - cos t
Acceleration a(t) = $\frac{dv}{dt}$
a(t) = sin t
When t = 0; x(t) = 0
When t = $\pi$; x(t) = $\pi$ > 0
When t = 0; x(t) = 2$\pi$ > 0
(b) Equation can be represented by
x(t) = sin t
v = $\frac{d}{dt}$ x(t) = cos t
As displacement and velocity are involving sin t and cos t hence these equations represent periodic.
Give example of a motion where x > 0, v < 0, a > 0 at a particular instant.
Let the motion is represented by
x(t) = A + Be-$\gamma$t ... (i)
$$ \begin{aligned} &\text{Let} A >B \text { and } \gamma>0 \\\\ &\text{Now velocity} \,x(t) =\frac{d x}{d t}=-B \gamma e^{-\gamma t} \\\\ &\text{Acceleration} \, a(t) =\frac{d x}{d t}=B \gamma^2 e^{-\gamma t} \end{aligned} $$
Suppose we are considering any instant $t$, then from Eq. (i), we can say that
$x(t)>0 ; v(t)<0$ and $a>0$
An object falling through a fluid is observed to have acceleration given by $a = g - bv$ where $g$ = gravitational acceleration and $b$ is constant. After a long time of release, it is observed to fall with constant speed. What must be the value of constant speed?
When speed becomes constant acceleration $a=\frac{d v}{d t}=0$
Given acceleration
$$ a=g-b v $$
where, $g=$ gravitational acceleration
Clearly, from above equation as speed increases acceleration will decrease. At a certain speed say $v_0$, acceleration will be zero and speed will remain constant.
Hence,
$$ \begin{aligned} &a =g-b v_0=0 \\\\ &v_0 =g / b \end{aligned} $$