A graph of x versus t is shown in figure. Choose correct alternatives given below

The particle was released from rest at t = 0
At B, the acceleration a > 0
Average velocity for the motion between A and D is positive
The speed at D exceeds that at Ε
For the one-dimensional motion, described by $x=t- \text{sin}t$
$x(t)>0$ for all $t>0$
$v(t)>0$ for all $t>0$
$a(t)>0$ for all $t>0$
$v(t)$ lies between $0$ and $2$
A spring with one end attached to a mass and the other to a rigid support is stretched and released.
Magnitude of acceleration, when just released is maximum
Magnitude of acceleration, when at equilibrium position, is maximum
Speed is maximum when mass is at equilibrium position
Magnitude of displacement is always maximum whenever speed is minimum
A ball is bouncing elastically with a speed 1 m/s between walls of a railway compartment of size 10 m in a direction perpendicular to walls. The train is moving at a constant velocity of 10 m/s parallel to the direction of motion of the ball. As seen from the ground:
The direction of motion of the ball changes every 10 s.
Speed of ball changes every 10 s.
Average speed of ball over any 20 s interval is fixed.
The acceleration of ball is the same as from the train.
Refer to the graph in figure. Match the following:
| Graph | Characteristics |
|---|---|
| (a) | (i) has v > 0 and a < 0 throughout |
| (b) | (ii) has x > 0 throughout and has a point with v = 0 and a point with a = 0 |
| (c) | (iii) has a point with zero displacement for t > 0 |
| (d) | (iv) has v < 0 and a > 0 |

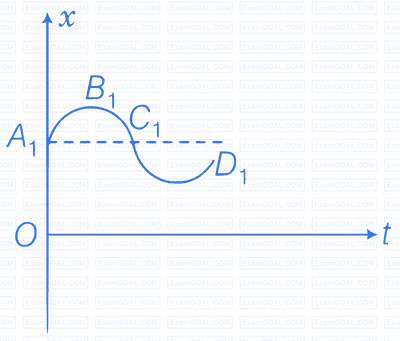


We have to analyze slope of each curve i.e., $\frac{dx}{dt}$. For peak points $\frac{dx}{dt}$ will be zero as x is maximum at peak points.
For graph (a), there is a point (B) for which displacement is zero. So, a matches with (iii)
In graph (b), x is positive (> 0) throughout and at point $B_1$, $v = \frac{dx}{dt}$ = 0. since, at point of curvature changes a = 0, So b matches with (ii)
In graph (c), slope $V = \frac{dx}{dt}$ is negative hence, velocity will be negative. so matches with (iv)
In graph (d), as slope $V = \frac{dx}{dt}$ is positive hence, V > 0
Hence, d matches with (i)