A particle falling vertically from a height hits a plane surface inclined to horizontal at an angle $\theta$ with speed $v_0$ and rebounds elastically. Find the distance along the plane where it will hit second time.

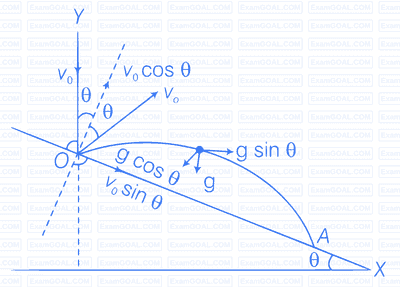
Considering x and y-axes as shown in the diagram. For the motion of the projectile from O to A.
$ y = 0, u_y = v_0 \cos \theta \\ a_y = -g \cos \theta, t = T $
Applying equation of kinematics,
$ y = u_y t + \frac{1}{2} a_y t^2 $
$ ⇒ \quad 0 = v_0 \cos \theta \; T + \frac{1}{2}(-g \cos \theta) T^2 $
$ ⇒ \quad T \left[ v_0 \cos \theta - \frac{g \cos \theta \; T}{2} \right] = 0 $
$ T = \frac{2 v_0 \cos \theta}{g \cos \theta} $
As $T = 0$, corresponds to point $O$
Hence,
$ T = \frac{2v_0}{g} $
Now considering motion along $OX$.
$ x = L, \ u_x = v_0 \sin \theta, \ a_x = g \sin \theta, \ t = T = \frac{2v_0}{g} $
Applying equation of kinematics,
$ x = u_x t + \frac{1}{2} a_x t^2 $
$ \Rightarrow L = v_0 \sin \theta \ t + \frac{1}{2} g \sin \theta \ t^2 = (v_0 \sin \theta) (T) + \frac{1}{2} g \sin \theta \ T^2 $
$ \qquad = (v_0 \sin \theta) \left( \frac{2v_0}{g} \right) + \frac{1}{2} g \sin \theta \times \left( \frac{2v_0}{g} \right)^2 $
$ \qquad = \frac{2v_0^2}{g} \sin \theta + \frac{1}{2} g \sin \theta \times \frac{4v_0^2}{g^2} = \frac{2v_0^2}{g} [\sin \theta + \sin \theta] $
$ \Rightarrow L = \frac{4v_0^2}{g} \sin \theta $
A girl riding a bicycle with a speed of 5 m/s towards north direction, observes rain falling vertically down. If she increases her speed to 10 m/s, rain appears to meet her at 45° to the vertical. What is the speed of the rain? In what direction does rain fall as observed by a ground-based observer?
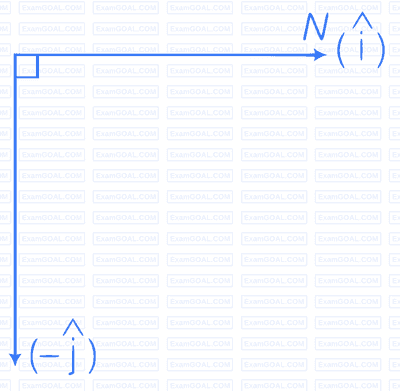
Assume north to be $ \hat{i} $ direction and vertically downward to be $-\hat{j} $.
Let the rain velocity $ \mathbf{v}_r $ be $ a \hat{i} + b \hat{j} $.
$\mathbf{v}_r = a \hat{i} + b \hat{j} $
Case I Given velocity of girl = $ \mathbf{v}_g = (5 \text{ m/s})\hat{i} $
Let $ \mathbf{v}_{rg} $ = Velocity of rain w.r.t girl
$ \mathbf{v}_{rg} = \mathbf{v}_r - \mathbf{v}_g = (a\hat{i} + b\hat{j}) - 5\hat{i} $
$ = (a - 5) \hat{i} + b \hat{j} $
According to question rain, appears to fall vertically downward. Hence,
$ a - 5 = 0 \Rightarrow a = 5 $
Case II Given velocity of the girl, $ \mathbf{v_g} = (10 \text{ m/s}) \hat{i} $
$ \mathbf{v_{rg}} = \mathbf{v_r} - \mathbf{v_g} $
$ = (a\hat{i} + b\hat{j}) - 10\hat{i} = (a - 10)\hat{i} + b\hat{j} $
According to question rain appears to fall at 45° to the vertical hence $ \tan 45° = \frac{b}{a - 10} = 1 $
$ \Rightarrow b = a - 10 = 5 - 10 = -5 $
Hence, velocity of rain $ = a\hat{i} + b\hat{j} $
$ \Rightarrow \mathbf{v_r} = 5\hat{i} - 5\hat{j} $
Speed of rain = $|\mathbf{v_r}| = \sqrt{(5)^2 + (-5)^2} = \sqrt{50} = 5\sqrt{2} \text{ m/s} $
A river is flowing due east with a speed 3 m/s. A swimmer can swim in still water at a speed of 4 m/s (figure).
(a) If swimmer starts swimming due north, what will be his resultant velocity (magnitude and direction)?
(b) If he wants to start from point A on south bank and reach opposite point B on north bank,
(i) which direction should he swim?
(ii) what will be his resultant speed?
(c) From two different cases as mentioned in (a) and (b) above, in which case will he reach opposite bank in shorter time?
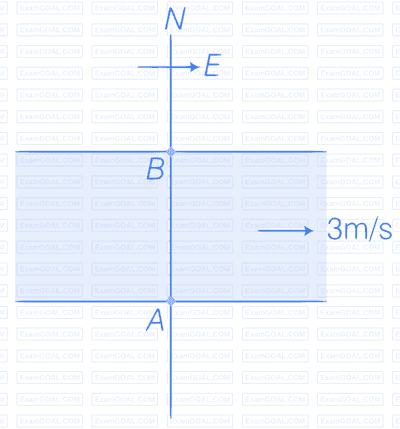
Given, Speed of the river $(v_r) = 3 \text{ m/s (east)}$
Speed of swimmer $(v_s) = 4 \text{ m/s (east)}$
(a) When swimmer starts swimming due north then his resultant velocity
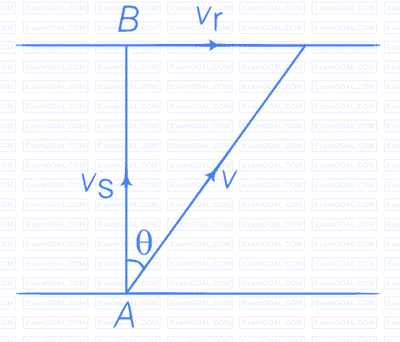
$ v = \sqrt{v_r^2 + v_s^2} = \sqrt{(3)^2 + (4)^2} $
$ = \sqrt{9 + 16} = \sqrt{25} = 5 \text{ m/s} $
$ \tan \theta = \frac{v_r}{v_s} = \frac{3}{4} $
$ = 0.75 = \tan 36^\circ 54' $
Hence,
$ \theta = 36^\circ 54' \text{ N} $
(b) To reach opposite points B, the swimmer should swim at an angle $ \theta $ of north. Resultant speed of the swimmer
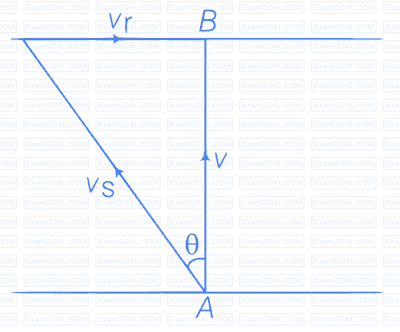
$ v = \sqrt{v_s^2 - v_r^2} = \sqrt{(4)^2 - (3)^2} \\ = \sqrt{16 - 9} = \sqrt{7} \text{ m/s} $
$ \tan \theta = \frac{v_r}{v} = \frac{3}{\sqrt{7}} $
$\Rightarrow \quad \theta = \tan^{-1} \left( \frac{3}{\sqrt{7}} \right) \text{ of north} $
(c) In case (a),
Time taken by the swimmer to cross the river, $ t_1 = \frac{d}{v_s} = \frac{d}{4} $
In case (b),
Time taken by the swimmer to cross the river
$ t_1 = \frac{d}{v} = \frac{d}{\sqrt{7}} $
As
$ \frac{d}{4} < \frac{d}{\sqrt{7}}, \text{ therefore } t_1 < t_2 $
Hence, the swimmer will cross the river in shorter time in case (a).
(a) the effective angle to the horizontal at which the ball is projected inair as seen by a spectator.
(b) what will be time of flight?
(c) what is the distance (horizontal range) from the point of projection at which the ball will land?
(d) find $\theta$ at which he should throw the ball that would maximise the horizontal range as found in (iii).
(e) how does $\theta$ for maximum range change if $u > u_0, u = u_0, u < v_0$?
(f) how does $\theta$ in (v) compare with that for $u = 0 \ (i.e., 45^\circ)$?
Consider the adjacent diagram.
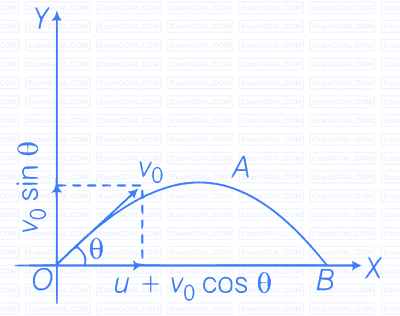
(a) Initial velocity in
x-direction,
$u_x = u + v_0 \cos\theta$
$u_y = \text{Initial velocity in y-direction}$
$= v_0 \sin\theta$
where angle of projection is $\theta$.
Now, we can write
$\tan\theta = \frac{u_y}{u_x} = \frac{v_0 \sin\theta}{u + v_0 \cos\theta}$
$\Rightarrow \theta = \tan^{-1} \left( \frac{v_0 \sin\theta}{u + v_0 \cos\theta} \right)$
(b) Let T be the time of flight.
As net displacement is zero over time period T.
$ y = 0,\ u_y = v_0 \sin \theta,\ a_y = -g,\ t = T $
We know that
$ y = u_y t + \frac{1}{2}a_y t^2 $
$ \Rightarrow \ 0 = v_0 \sin \theta \ T + \frac{1}{2}\ (-g)\ T^2 $
$ \Rightarrow \ T \left[ v_0 \sin \theta - \frac{g}{2} T \right] = 0 \ \Rightarrow \ \ T = 0, \frac{2v_0 \sin \theta}{g} $
$ T=0_1, \ \text{corresponds to point O.} $
Hence,$ T = \frac{2u_0 \sin \theta}{g} $
(c) Horizontal range, $ R = (u + v_0 \cos \theta) T = (u + v_0 \cos \theta) \frac{2v_0 \sin \theta}{g} $
$ = \frac{v_0}{g} \left[ 2u \sin \theta + v_0 \sin 2\theta \right] $
(d) For horizontal range to be maximum, $ \frac{dR}{d\theta} = 0 $
$ \Rightarrow \quad \frac{v_0}{g} \left[ 2u \cos \theta + v_0 \cos 2\theta \times 2 \right] = 0 $
$ \Rightarrow \quad 2u \cos \theta + 2v_0 \left[ 2\cos^2 \theta - 1 \right] = 0 $
$ \Rightarrow \quad 4v_0 \cos^2 \theta + 2u \cos \theta - 2v_0 = 0 $
$ \Rightarrow \quad 2v_0 \cos^2 \theta + u \cos \theta - v_0 = 0 $
$ \Rightarrow \quad \cos \theta = \frac{-u \pm \sqrt{u^2 + 8v_0^2}}{4v_0} $
$ \Rightarrow \quad \theta_{\text{max}} = \cos^{-1} \left[ \frac{-u \pm \sqrt{u^2 + 8v_0^2}}{4v_0} \right] $
(e) If $ u = v_0 $,
$ \cos \theta = \frac{-v_0 \pm \sqrt{v_0^2 + 8v_0^2}}{4v_0} = \frac{-1 + 3}{4} = \frac{1}{2} $
$ \theta = 60^\circ $
$ \Rightarrow $ If $ u \ll v_0 $, then $ 8v_0^2 + u^2 \approx 8v_0^2 $
$ \theta_{\max} = \cos^{-1} \left[ \frac{-u + 2 \sqrt{2}v_0}{4v_0} \right] = \cos^{-1} \left[ \frac{1}{\sqrt{2}} - \frac{u}{4v_0} \right] $
If $ u \ll v_0 $, then
$ \theta_{\max} = \cos^{-1} \left( \frac{1}{\sqrt{2}} \right) = \frac{\pi}{4} $
If $ u > u_0 $ and $ u \gg v_0 $
$ \theta_{\max} = \cos^{-1} \left[ \frac{-u + u}{4v_0} \right] = 0 \Rightarrow \theta_{\max} = \frac{\pi}{2} $
(f) If $ u = 0 $, $ \theta_{\max} = \cos^{-1} \left[ \frac{0 \pm \sqrt{8v_0^2}}{4v_0} \right] = \cos^{-1} \left( \frac{1}{\sqrt{2}} \right) = 45^\circ $
Motion in two dimensions, in a plane can be studied by expressing position, velocity and acceleration as vectors in cartesian coordinates $ \mathbf{A} = A_x \hat{i} + A_y \hat{j} $, where $ \hat{i} $ and $ \hat{j} $ are unit vector along x and y-directions, respectively and $ A_x $ and $ A_y $ are corresponding components of $ \mathbf{A} $. Motion can also be studied by expressing vectors in circular polar coordinates as $ \mathbf{A} = A_r \hat{r} + A_\theta \hat{\theta} $, where $ \hat{r} = \frac{\mathbf{r}}{r} = \cos{\theta} \hat{i} + \sin{\theta} \hat{j} $ and $ \hat{\theta} = -\sin{\theta} \hat{i} + \cos{\theta} \hat{j} $ are unit vectors along direction in which r and $ \theta $ are increasing.
(a) Express $ \hat{i} $ and $ \hat{j} $ in terms of $ \hat{r} $ and $ \hat{\theta} $.
(b) Show that both $ \hat{r} $ and $ \hat{\theta} $ are unit vectors and are perpendicular to each other.
(c) Show that $ \frac{d}{dt} ( \hat{r}) = \omega \hat{\theta} $, where $ \omega = \frac{d\theta}{dt} $ and $ \frac{d}{dt} ( \hat{\theta}) = - \omega \hat{r} $.
(d) For a particle moving along a spiral given by $ r = a \theta \hat{r} $, where $ a = 1 $ (unit), find dimensions of a.
(e) Find velocity and acceleration in polar vector representation for particle moving along spiral described in (d) above.
(a) Given, unit vector
$ \hat{r} = \cos \theta \hat{i} + \sin \theta \hat{j} $ $ \hspace{2cm} \text{...(i)}$
$ \hat{\theta} = -\sin \theta \hat{i} + \cos \theta \hat{j} $$ \hspace{1.5cm} \text{...(ii)}$
Multiplying Eq. (i) by $ \sin \theta $ and Eq. (ii) with $ \cos \theta $ and adding
$ \hat{r} \sin \theta + \hat{\theta} \cos \theta = \sin \theta \cos \theta \hat{i} + \sin^2 \theta \hat{j} + \cos^2 \theta \hat{j} - \sin \theta \cos \theta \hat{i} $
$ = \hat{j} (\cos^2 \theta + \sin^2 \theta) = \hat{j} $
$ \Rightarrow \hat{r} \sin \theta + \hat{\theta} \cos \theta = \hat{j} $
By Eq. (i) $ \times \cos \theta - $ Eq. (ii) $ \times \sin \theta $
$ n (\hat{r} \cos \theta - \hat{\theta} \sin \theta) = \hat{i} $
(b) $ \mathbf{\hat{r}} \cdot \mathbf{\hat{\theta}} = (\cos \theta\; \mathbf{\hat{i}} + \sin \theta\; \mathbf{\hat{j}}) \cdot (- \sin \theta\; \mathbf{\hat{i}} + \cos \theta\; \mathbf{\hat{j}}) = - \cos \theta \cdot \sin \theta + \sin \theta \cdot \cos \theta = 0 $
$ \Rightarrow \theta = 90^\circ \; \text{Angle between} \; \mathbf{\hat{r}} \; \text{and} \; \mathbf{\hat{\theta}}. $
(c) Given, $ \mathbf{\hat{r}} = \cos \theta\; \mathbf{\hat{i}} + \sin \theta\; \mathbf{\hat{j}} $
$ \frac{d\mathbf{\hat{r}}}{dt} = \frac{d}{dt}(\cos \theta\; \mathbf{\hat{i}} + \sin \theta\; \mathbf{\hat{j}}) = - \sin \theta \cdot \frac{d\theta}{dt}\; \mathbf{\hat{i}} + \cos \theta \cdot \frac{d\theta}{dt}\; \mathbf{\hat{j}} $
$ = \omega [-\sin \theta\; \mathbf{\hat{i}} + \cos \theta\; \mathbf{\hat{j}}] $
$ \left(\therefore \; \theta = \frac{d\theta}{dt} \right) $
(d) Given, $ \mathbf{r} = a\theta\mathbf{\hat{r}}, $ here, writing dimensions $[\mathbf{r}] = [a][\theta][\mathbf{\hat{r}}] $
$ \Rightarrow L = [a] \% 1 \Rightarrow [a] = L = [M^0L^1T^0] $
(e) Given, $ a = 1 $ unit
$ r = \theta \hat{\mathbf{r}} = \theta [\cos \theta \hat{\mathbf{i}} + \sin \theta \hat{\mathbf{j}}] $
Velocity,
$ \mathbf{v} = \frac{d\mathbf{r}}{dt} = \frac{d\theta}{dt} \hat{\mathbf{r}} + \theta \frac{d}{dt} \hat{\mathbf{r}} = \frac{d\theta}{dt} \hat{\mathbf{r}} + \theta \frac{d}{dt} [(\cos \theta \hat{\mathbf{i}} + \sin \theta \hat{\mathbf{j}})] $
$ = \frac{d\theta}{dt} \hat{\mathbf{r}} + \theta \left[ (-\sin \theta \hat{\mathbf{i}} + \cos \theta \hat{\mathbf{j}}) \frac{d\theta}{dt} \right] $
$ = \frac{d\theta}{dt} \hat{\mathbf{r}} + \theta \hat{\mathbf{\theta}} = \omega \hat{\mathbf{r}} + \omega \hat{\mathbf{\theta}} $
Acceleration,
$ \mathbf{a} = \frac{d}{dt} [\omega \hat{\mathbf{r}} + \omega \theta\hat{\mathbf{\theta}}] = \frac{d}{dt} \left[ \frac{d\theta}{dt} \hat{\mathbf{r}} + \frac{d\theta}{dt} (\theta \hat{\mathbf{\theta}}) \right] $
$ = \frac{d^2\theta}{dt^2} \hat{\mathbf{r}} + \frac{d\theta}{dt} \cdot \frac{d\hat{\mathbf{r}}}{dt} + \frac{d^2\theta}{dt^2} \hat{\mathbf{\theta}} + \omega \frac{d}{dt} (\theta \hat{\mathbf{\theta}}) $
$ = \frac{d^2\theta}{dt^2} \hat{\mathbf{r}} + \omega [-\sin \theta \hat{\mathbf{i}} + \sin \theta \hat{\mathbf{j}}] + \frac{d^2\theta}{dt^2} \hat{\mathbf{\theta}} + \omega \frac{d}{dt} (\theta \hat{\mathbf{\theta}}) $
$ = \frac{d^2\theta}{dt^2} \hat{\mathbf{r}} + \omega^2 \hat{\mathbf{\theta}} + \frac{d^2\theta}{dt^2} \times \hat{\mathbf{\theta}} + \omega^2 \hat{\mathbf{\theta}} + \omega^2 (\theta \hat{\mathbf{r}}) $
$ = \left( \frac{d^2\theta}{dt^2} - \omega^2 \right) \hat{\mathbf{r}} + \left(2\omega^2 + \frac{d^2\theta}{dt^2} \theta \right) \hat{\mathbf{\theta}} $