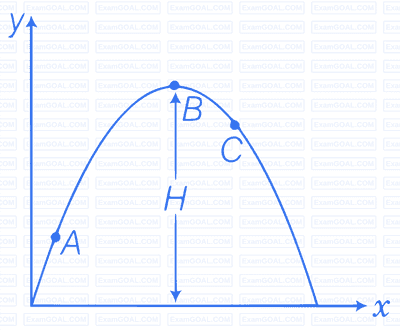
A particle is projected in air at some angle to the horizontal, moves along parabola as shown in figure where x and y indicate horizontal and vertical directions, respectively. Shown in the diagram, direction of velocity and acceleration at points A, B and C.
Consider the adjacent diagram in which a particle is projected at an angle $ \theta $.

$ v_x = \text{Horizontal component of velocity} = v \cos \theta = \text{constant.} $
$ v_y = \text{Vertical component of velocity} = v \sin \theta $
Velocity will always be tangential to the curve in the direction of motion and acceleration is always vertically downward and is equal to $ g $ (acceleration due to gravity).
A ball is thrown from a roof top at an angle of 45° above the horizontal. It hits the ground a few seconds later. At what point during its motion, does the ball have
(a) greatest speed
(b) smallest speed
(c) greatest acceleration
Explain.

Consider the adjacent diagram in which a ball is projected from point $ O $, and covering the path $ OABC $.
(a) At point $ B $, it will gain the same speed $ u $ and after that speed increases and will be maximum just before reaching $ C $.
(b) During upward journey from $ O $ to $ A $ speed decreases and will be minimum at point $ A $.
(c) Acceleration is always constant throughout the journey and is vertically downward equal to $ g $.
A football is kicked into the air vertically upwards. What is its (a) acceleration and (b) velocity at the highest point?
(a) Consider the adjacent diagram in which a football is kicked into the air vertically upwards. Acceleration of the football will always be vertical downward and is equal to g.
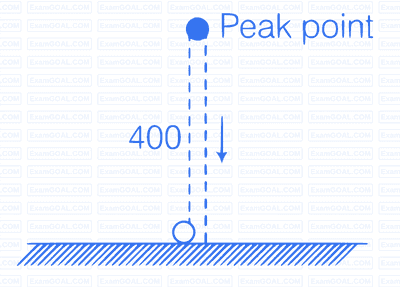
(b) When the football reaches the highest point velocity will be zero as it is continuously retarded by acceleration due to gravity g.
A, B and C are three non-collinear, non co-planar vectors. What can you say about direction of $A \times (B \times C)$?
The direction of $(\mathbf{B} \times \mathbf{C})$ will be perpendicular to the plane containing $\mathbf{B}$ and $\mathbf{C}$ by right hand rule. $\mathbf{A} \times (\mathbf{B} \times \mathbf{C})$ will lie in the plane of $\mathbf{B}$ and $\mathbf{C}$ and is perpendicular to vector $\mathbf{A}$.
A boy travelling in an open car moving on a levelled road with constant speed tosses a ball vertically up in the air and catches it back. Sketch the motion of the ball as observed by a boy standing on the footpath. Give explanation to support your diagram.
The path of the ball observed by a boy standing on the footpath is parabolic. The horizontal speed of the ball is same as that of the car, therefore, ball as well as car travels equal horizontal distance. Due to its vertical speed, the ball follows a parabolic path.

Note: We must be very clear that we are working with respect to ground. When we observe with respect to the car motion, it will be along the vertical direction only.