Following are four different relations about displacement, velocity and acceleration for the motion of a particle in general. Choose the incorrect one(s).
$v_{av} = \frac{1}{2}\left[ v(t_1) + v(t_2) \right]$
$v_{av} = \frac{r(t_2) - r(t_1)}{t_2 - t_1}$
$r = \frac{1}{2} \left( v(t_2) - v(t_1) \right) (t_2 - t_1)$
$a_{av} = \frac{v(t_2) - v(t_1)}{t_2 - t_1}$
For a particle performing uniform circular motion, choose the correct statement(s) from the following.
Magnitude of particle velocity (speed) remains constant
Particle velocity remains directed perpendicular to radius vector
Direction of acceleration keeps changing as particle moves
Angular momentum is constant in magnitude but direction keeps changing
For two vectors A and B, |A + B| = |A − B| is always true when
|A| = |B| ≠ 0
A ⊥ B
|A| = |B| ≠ 0 and A and B are parallel or anti-parallel
when either |A| or |B| is zero
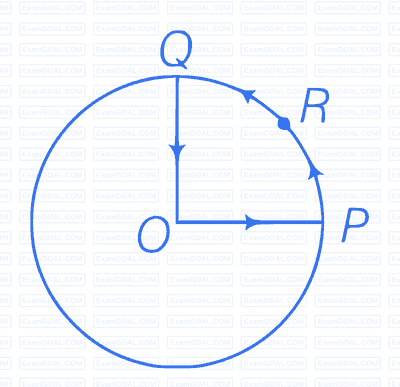
A cyclist starts from centre O of a circular park of radius 1 km and moves along the path OPRQO as shown in figure. If he maintains constant speed of $10 \, \text{ms}^{-1}$, what is his acceleration at point R in magnitude and direction?

As shown in the adjacent figure, the cyclist covers the path OPRQO. As we know whenever an object performing circular motion, acceleration is called centripetal acceleration and is always directed towards the centre.
Hence, acceleration at R is $a = \frac{v^2}{r}$
⇒ $a = \frac{(10)^2}{1 \, \text{km}} = \frac{100}{10^3} = 0.1 \, \text{m/s}^2$ along RO.
A particle is projected in air at some angle to the horizontal, moves along parabola as shown in figure where x and y indicate horizontal and vertical directions, respectively. Shown in the diagram, direction of velocity and acceleration at points A, B and C.
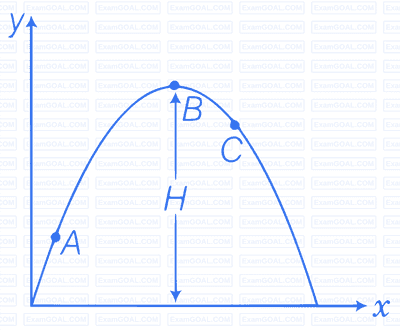
Consider the adjacent diagram in which a particle is projected at an angle $ \theta $.

$ v_x = \text{Horizontal component of velocity} = v \cos \theta = \text{constant.} $
$ v_y = \text{Vertical component of velocity} = v \sin \theta $
Velocity will always be tangential to the curve in the direction of motion and acceleration is always vertically downward and is equal to $ g $ (acceleration due to gravity).