In a diatomic molecule, the rotational energy at a given temperature
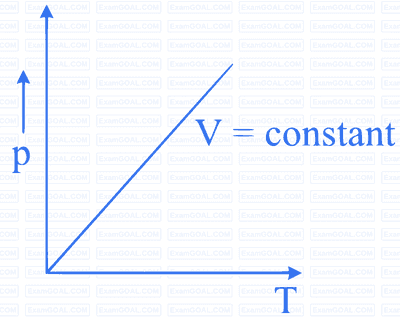
Which of the following diagrams (figure) depicts ideal gas behaviour?



When an ideal gas is compressed adiabatically, its temperature rises the molecules on the average have more kinetic energy than before. The kinetic energy increases,
Calculate the number of atoms in 39.4 g gold. Molar mass of gold is $197 \mathrm{~g} \mathrm{~mole}^{-1}$.
$$ \begin{aligned} \text { Molar mass } & =\text { Mass of Avogadro's number of atoms (Molecules). } \\ & =6.023 \times 10^{23} \text { atoms. } \end{aligned}$$
Given, $\quad$ molar mass of gold $=197 \mathrm{~g} / \mathrm{mol}$
Now,
$\therefore \quad 197 \mathrm{~g}$ of gold contains $=6.023 \times 10^{23}$ atoms
$\therefore \quad 1 \mathrm{~g}$ of gold contain $=\frac{6.023 \times 10^{23}}{197}$ atoms
$\therefore \quad 39.4 \mathrm{~g}$ of gold contains $=\frac{6.023 \times 10^{23} \times 39.4}{197} \Rightarrow 1.20 \times 10^{23}$ atoms
The volume of a given mass of a gas at $27^{\circ} \mathrm{C}, 1 \mathrm{~atm}$ is 100 cc . What will be its volume at $327^{\circ} \mathrm{C}$ ?
We have to convert the given temperatures in kelvin .
If pressure of a given mass of the gas is kept constant, then
$$\begin{array}{ll} & V \propto T \\ \Rightarrow & \frac{V}{T}=\text { constant } \quad \left[\begin{array}{l} V=\text { Volume of gas } \\ T=\text { Temperature of gas } \end{array}\right]\\ \Rightarrow & \frac{V_1}{T_1}=\frac{V_2}{T_2} \\ \Rightarrow & V_2=V_1\left(\frac{T_2}{T_1}\right) \\ & T_1=273+27=300 \mathrm{~K} \\ & T_2=273+327=600 \mathrm{~K} \\ \text { But } & V_1=100 \mathrm{cc} \\ & V_2=V_1\left(\frac{600}{300}\right) \\ & V_2=2 V_1 \\ \therefore \quad & V_2=2 \times 100=200 \mathrm{cc} \end{array}$$